

Mechanics of Waves
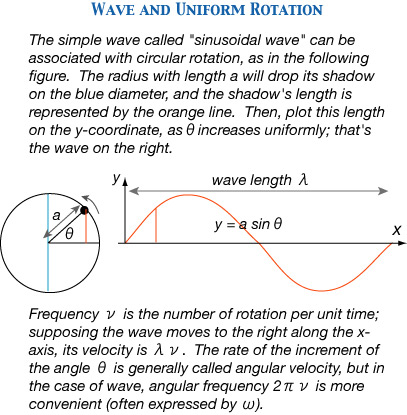
In order to understand the elements of quantum mechanics, you need to know the basic features of waves, as treated in classical mechanics. Its mathematics is not too hard. We will present the simplest case (assuming that the angle Æ increases uniformly with time, and omitting the time variable t). Any mechanical system (not necessarily a 'wave' on appearence) with this sinusoidal oscillation is what Tomonaga called "Planck's oscillator" (Tomonaga 1969, p. 92).
Various waves can be combined to form one complex wave. This process is called superposition, and this plays a crucial role in quantum mechanics, as we will see later. Superposition itself is quite easy to understand. As you can intuitively see from the following figure, the resulting new wave is obtained simply by adding two waves (in technical words, linear combination).
With this preparation, you can begin to understand de Broglie's idea. Einstein has shown that light can be regarded as a particle, at least in certain contexts; but we have a longstanding tradition of treating light as a wave. In particular, Young's famous 2-slit experiment seems to be explained only by the wave theory. This means we have a dual picture of light, one as a particle, another as a wave.
Drawing on this duality, De Broglie thought that the motion of a particle may also be treated as a wave, satisfying a certain wave equation; thus not only light, but matter may have the same sort of duality. This idea has an immediate implication on the trajectory of an electron (it is a kind of matter) around the nucleus: Why does that trajectory have to have some integral number related to quantum? If the electron may be regarded as a wave, it is quite natural, for being stable, the trajectory must have a length which is the proper wave length multiplied by an integral number! (In the following figure, the radius is represented by r, not a, because r is the radius of the trajectory, not of the circle associated with the wave.)
Setting aside the harder part of mathematics, we will mention only that de Broglie tried to utilize what Einstein has shown as regards photon: the relationship between energy E and momentum p on the one hand, and frequency and wavelength on the other.
E = hË
p = h/ É
By this relationship, we can bridge a gap between the particulate picture and the wave picture of the matter. This was a quite important step for the development of quantum mechanics. However, notice that de Broglie's "wave" is still a wave within space and time. Although this image worked for treating atomic structure and other phenomena, it will turn out to be untenable for the general quantum problem. You should be very careful not to maintain this image, when it comes to Schroedinger's wave equation.
De Broglie's idea was propounded in his doctrate thesis (1924), and he was awarded the Nobel Prize (physics, 1929) for this. Schroedinger was stimulated by de Broglie's work, and worked out his own generalization, i.e., the famous wave equation. Anyway, that free electrons behave both as a particle and as a wave, was later experimentally confirmed by Davisson and Germer in the United States (1927), as was explained by Dr. Tomonaga (1997, 11-15).
Bohr, N. (1934), Atomic Theory and the Description of Nature, Ox Bow Press (reprint)
Tomonaga, S. (1969) ÊqÍw IiæQÅjAÝ·¸[B
Tomonaga, S. (1997) ÊqÍw IIiæQÅjAÝ·¸[B
See also a useful site on Microphysics, at Kyushu University: http://www2.kutl.kyushu-u.ac.jp/seminar/MicroWorld/MicroWorld.html