

Einstein-Podolsky-Rosen Paradox

In 1935, the paper by Einstein, Podolsky, and Rosen appeared in the Physical Review 47, and this came as "a bolt from the blue" to the Bohr camp. Bohr's collaborator L. Rosenfeld reports: "as soon as Bohr had heard my report of Einstein's argument, everything else was abandoned: we had to clear up such a misunderstanding at once" (Wheeler and Zurek 1983, 142). And they finished their reply in less than 6 weeks. However, in Bohr's contribution to the Einstein volume (of the Living Philosophers), Bohr gives no hint of such an excitement. But it is quite important to know the essential part of Einstein-Podolsky-Rosen paper, now often referred to as the EPR Paradox.
Their paper begins with two definitions, one for a "complete theory", another for "physically real".
Whatever the meaning assigned to the term complete, the following requirement for a complete theory seems to be a necessary one: every element of the physical reality must have a counterpart in the physical theory. (Wheeler and Zurek 1983, 138)
If, without in any way disturbing a system, we can predict with certainty (i.e., with probability equal to unity) the value of a physical quantity, then there exists an element of physical reality corresponding to this physical quantity. (ibid.)
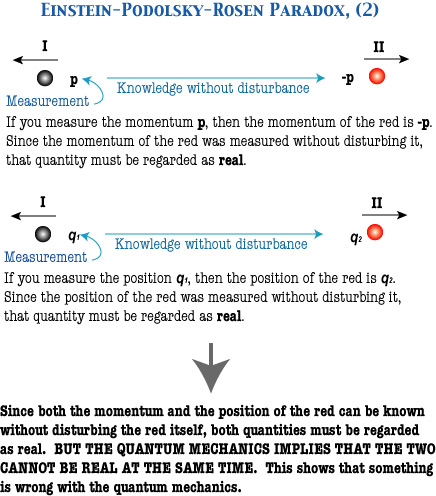
Thus they prepared the ground for their onslaught on the quantum mechanics. Their argument goes as follows: Suppose the quantum mechanics is complete in this sense. Then, if, for instance, the momentum of a particle is known, its position (coordinate) has no physical reality; thus we should be unable to attribute reality to both of them. However, we have devised a Gedankenexperiment in which we can attribute reality to both the momentum and the position (coordinate) of a particle, according to the second definition. Thus both are real in our sense, but the quantum mechanics fails to account for this. And this shows that it cannot be complete in the first sense. Here are a couple of pictures illustrating the essential part of their argument.
Suppose we prepare the system consisting of two particles, initially interacting with each other but separated far away after that. However, by the help of measurement of one, we can know the state of the other. Here, the point is that this measurement is done when the two cannot interact because of a great separation.
Then, what is going to happen when the measurement is made? We can choose what is to be measured, but in each case, the measurement of a particle gives sure information as regards the other, without disturbing the latter.
Bohr is to reply in the next issue of the Physical Review, but we can point out at least this much. As we have already seen, Bohr frequently emphasized that "because of interaction between the object and the agency of observation", there appears indeterminacy characteristic of the quantum phenomena. The EPR paper contributed greatly to clarifying the ambiguity of this "interaction", for which Bohr was mainly responsible.
Incidentally, this feature of quantum mechanics, i.e. that we can get information of the state of a part by measuring another part of the system, is closely related with the possibility of the quantum computer, now studied extensively by a number of physicists and engineers.
Bohr, N. (1958) Essays 1933-1957 On Atomic Physics and Human Knowledge
Tomonaga, S. (1997) 量子力学 II(第2版)、みすず書房。
Wheeler, J. A., and Zurek, W. H., ed. (1983) Quantum Theory and Measurement, Princeton University Press.
See also a useful site on Microphysics, at Kyushu University: http://www2.kutl.kyushu-u.ac.jp/seminar/MicroWorld/MicroWorld.html