

Introduction to the Philosophy of Space and Time
How to Convert Mass into Length?
In Schwarzschild Geometry, we expressed mass M in unit of length. That made physics much easier and clearer than otherwise. But you may wonder how? I will sketch the procedure in the following.
First, you should recall Newton's 2nd law of motion:
(1) F = Ma
Here, F is force, M is mass, and a is acceleration. But what is acceleration, do you remember? It is the rate of the change of the velocity, and the latter was expressed in the unit: (meter/second), (m/s) for short, if we use the familiar system of quantities in physics. All right, then, acceleration should be in terms of (m/ss), since it is the 1st derivative of the velocity, and hence time variable must be squared. But what is the unit for the mass? There are many alternatives, but let us adopt kilogram which is quite in conformity with the preceding system. Then, the force is now expressed in terms of the unit: (kilogram・(m/ss)). In physics, the unit force which gives the acceleration 1 (m/ss) to the mass of 1 kilogram is called 1 Newton.
Next, let us recall Newton's law of gravitation:
(2) F = G・(MM'/rr)
where r means the distance between the two particles with mass M and M', and G is the constant of gravity. If mass is in terms of kilogram and distance in terms of meter, then the unit of this force must be: (kilogram・(m/ss)). From this, it follows that G is expressed in terms of the unit: (mmm/kilogram・ss). The cube of m, because the m of F must be multiplied by the square of r (hence mm) on the righthand side of (2).
In order to convert mass into units of length, we use this constant G together with the velocity of light c, as was already done when we converted time into units of length. Now, In terms of kilogram, meter, and second, G has the following experimentally determined value [unit is (mmm/kilogram・ss)]:
(3) G = 6.6726/100000000000
Then, recall that the square of c appeared when we converted Einstein's famous equation for the equivalence between energy and mass,
E = mcc .
So, again, we need the square of c, in order to convert mass into length. Specifically, we need G/cc, which is expressed in terms of m/kg (so that if we multiply a mass in kilograms by this factor, we can obtain the same mass in meters!). See the following calculation:
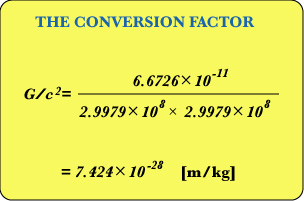
Thus we have done it! Take any mass expressed in terms of kilogram; then multiply it by this conversion factor, and the result is the mass in units of length, meters!
Last modified Nov. 14, 2004. (c) S. Uchii